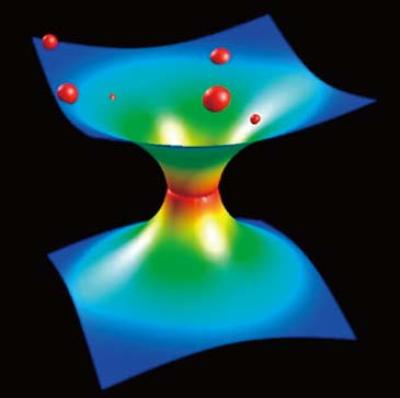
In the past few hundred years, scientists have searched for fundamental laws of nature by exploring phenomena at shorter and shorter distances. Does this progression continue indefinitely? Surprisingly, there are reasons to think that the hierarchical structure of nature will terminate at 10−35 meter, the so-called Planck length. Let us perform a thought-experiment to explain why this might be the case. Physicists build particle colliders to probe short distances. The more energy we use to collide particles, the shorter distances we can explore. This has been the case so far. One may then ask: can we build a collider with energy so high that it can probe distances shorter than the Planck length? The answer is no. When we collide particles with such high energy, a black hole will form and its event horizon will conceal the entire interaction area. Stated in another way, the measurement at this energy would perturb the geometry so much that the fabric of space and time would be torn apart. This would prevent physicists from ever seeing what is happening at distances shorter than the Planck length. This is a new kind of uncertainty principle. The Planck length is truly fundamental since it is the distance where the hierarchical structure of nature will terminate.
Space and time do not exist beyond the Planck scale, and they should emerge from a more fundamental structure. Superstring theory is a leading candidate for a mathematical framework to describe physics at the Planck scale since it contains all the ingredients necessary to unify general relativity and quantum mechanics and to deduce the Standard Model of particle physics. Superstring theory has helped us solve various mysteries of quantum gravity such as the information paradox of black holes posed by Stephen Hawking. The theory has given us insights into early universe cosmology and models beyond the Standard Model of particle physics. It provides powerful tools to study many difficult problems in theoretical physics—often involving strongly interacting systems—such as QCD (theory of quark interactions), quantum liquid and quantum phase transitions. It has also inspired many important developments in mathematics. All of these aspects of string theory are vigorously investigated at Kavli IPMU. (Last update: 2018/04/26)
Members
- Konstantin Aleshkin
- Robert Brandenberger
- Sunjin Choi
- Ioana Coman Lohi
- Vaibhav Gautam
- Simeon Hellerman
- Kentaro Hori
- Sagharsadat Hosseinisemnani
- Sergey Ketov
- Luca Marchetti
- Yuan Miao
- Todor Milanov
- Hayato Morimura
- David Morrison
- Shinji Mukohyama
- Hirosi Ooguri
- Jiaxin Qiao
- Shigeki Sugimoto
- Yuji Tachikawa
- Tadashi Takayanagi
- Victoria Venken
- Masataka Watanabe
- Taizan Watari
- Masahito Yamazaki
- Hao Zhang
- Yi Zhang






