November 28, 2024
Kavli Institute for the Physics and Mathematics of the Universe (Kavli IPMU, WPI)
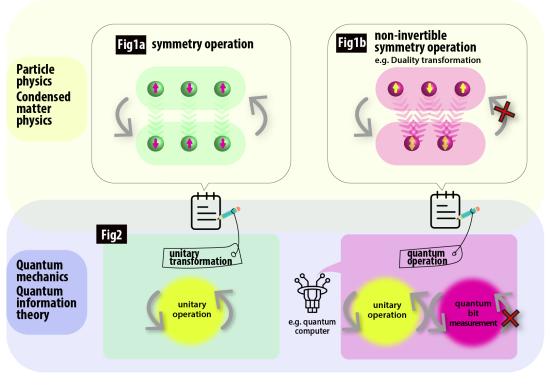
Theoretical physicists have established a close connection between the two rapidly developing fields in theoretical physics, quantum information theory and non-invertible symmetries in particle and condensed matter theories, after proving that any non-invertible symmetry operation in theoretical physics is a quantum operation, reports a recent study published in Physical Review Letters as an Editors’ Suggestion on November 6.
In physics, symmetry provides an important clue to the properties of a theory. For example, if the N-poles in a magnetic field are replaced by the S-poles, and the S-poles by the N-poles all at once, the forces on objects and the energy stored in the magnetic field remain the same, even though the direction of the magnetic field has now become reversed. This is because the equations describing the magnetic field are symmetric with respect to the operation of swapping the N and S poles.
Over the past few years, the concept of symmetries has received generalization in various directions in the theoretical study of particle physics and condensed matter physics, becoming an active area of research. One such generalization is non-invertible symmetry. The operation of conventional symmetries is always invertible. There exists a reverse operation to undone it. Non-invertible symmetry, on the other hand, allows certain non-invertibility in such symmetry operations.
Another field increasingly getting the attention of physicists in recent years has been quantum information theory. It is the theory that makes up the foundation of quantum computers.
A fundamental concept in quantum computation is performing various operations on memories called quantum bits, also known as qubits. Among them, any operation which can be undone is formulated by a mathematical operation called a unitary transformation. However, non-invertible operations, where there is no reverse operation, are also important, such as the measurement of quantum bits. These operations are carried out using a generalized concept of unitary transformation, called a quantum operation.
Several years ago, mathematician Marcel Bischoff and his collaborators had suggested an idea that the operation of non-invertible symmetries is a quantum operation. However, their idea was described in a framework applicable only to physical systems with specific properties, and unfamiliar to most of physics communities.
Now, a pair of researchers, The University of Tokyo School of Science graduate student Masaki Okada and The University of Tokyo Kavli Institute for the Physics and Mathematics of the Universe (Kavli IPMU, WPI) Professor Yuji Tachikawa, have shown that this idea can be nicely proved in a framework widely used in particle physics and condensed matter physics.
Until now, no one had known what a general property of non-invertible symmetry operations was. Okada and Tachikawa’s work has provided an answer that any operation of non-invertible symmetries is a quantum operation.
Paper details
Journal: Physical Review Letters
Paper title: Non-invertible symmetries act locally by quantum operations
Authors: Masaki Okada (1), Yuji Tachikawa (1)
Author affiliations:
1. Kavli Institute for the Physics and Mathematics of the Universe (WPI), University of Tokyo, Kashiwa, Chiba 277-8583, Japan
DOI: 10.1103/PhysRevLett.133.191602 (published November 6, 2024)
Paper abstract (Physical Review Letters)
Pre-print (arXiv.org)
Research contact
Yuji Tachikawa
Professor
Kavli Institute for the Physics and Mathematics of the Universe (Kavli IPMU, WPI)
E-mail: yuji.tachikawa_at_ipmu.jp
* Please change _at_ to @
Media contact
Motoko Kakubayashi
Press officer
Kavli Institute for the Physics and Mathematics of the Universe (Kavli IPMU, WPI)
E-mail: press_at_ipmu.jp
* Please change _at_ to @






